Prediction of motion of masses in a more complex pulley/mass assembly.
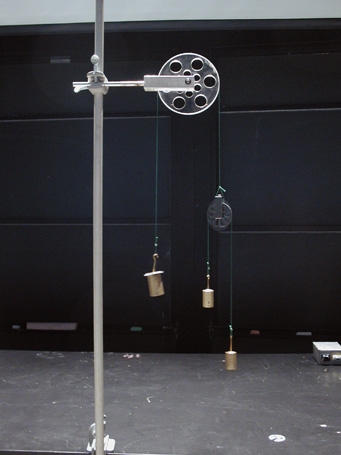
What it shows: This compund Atwood's Machine demonstrates an old and interesting problem. The two small weights on the right side are not of equal mass — one is 100 g and the other is 200 g. On the left side is a large mass equal to 300+ g (the extra + is the weight of the small pulley on the right side, about 21 g). If one ties together the two small weights on the right side, the apparatus will hang in stable equilibrium — the mass on the left side balances the masses on the right side. Furthermore, if the large weight on the left is given a modest push in either the upward or downward direction, it will continue to move in that direction at a constant speed, demonstrating that there is no net force acting on it. Now comes the puzzler: If one burns the string holding the two small weights together, what will be the ensuing motion of the system? Obviously the 200 g mass will accelerate downward, pulling the 100 g mass upward. But what about the 300+ g mass? Will it (a) move upward, (b) move downward, or (c) remain where it is? Discuss.
How it works: The experiment agrees with the mathematical analysis:1 both the 200 g and 300+ g mass move downward — the center-of-mass of the entire system accelerates down! You can arrive at this result with a thought experiment: imagine what would happen if the difference between the two masses on the right becomes extreme. Instead of 100 and 200 grams, suppose they're 5 and 295 grams. The tension in the string that accelerates the 5 g mass upwards will be VERY small (compared to the original case). Consequently, the tension of the string supporting the pulley will also be small and thus the weight on the left will fall down.
Setting it up: Attach to lecture bench-top with bench clamp as seen in photo. Use the small butane torch to burn the string. Video display is suggested.
Comments: The double Atwood's Machine problem demonstrates that equal masses don't always balance — a great puzzler to ponder!
Footnotes:
1. R. Newburgh, J. Peidle, and W. Rueckner, "When equal masses don't balance," Physics Education 39(3), 289-293 (2004).
2. also see R.L. Coelho, P.F. Borges, and R. Karam, "Atwood and Poggendorff: an insightful analogy," European Journal of Physics, Sept. 22, 2016. The Poggendorff balance would be a great demonstration to accompany the compound Atwood Machine.