What it shows:  The wave nature of light limits our ability to see the very small. Application of the Rayleigh limit of resolution tells us that the size of the smallest objects one can resolve under a microscope is approximately equal to the wavelength of light. The optical limits of a microscope are demonstrated as one attempts to resolve 1 μm diameter spheres (about twice the wavelength of light) — one sees spots of light surrounded by diffraction rings rather than sharply defined spheres, similar to the 3rd image (from: Cagnet/Francon/Thrierr, Atlas of Optical Phenomena).

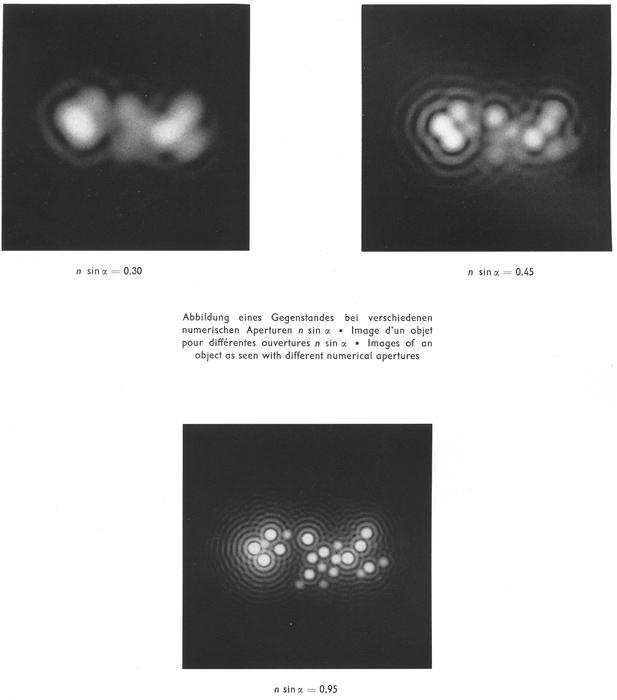
How it works:  The angular resolution, as given by the Rayleigh criterion, is \(\theta = 1.22 {\lambda \over a}\), where a is the aperture of the microscope (objective lens). The object under magnification is located just inside the focal length, f, of the objective lens. If we let the size of the object be x, then the object subtends an angle \(\theta = {x\over f}\) as seen from the objective. Equating these two angles gives us that \(x = 1.22{f \over a}{\lambda}\). Now, f/a is the "f-stop" and is a number close to 1 for the best lenses. Thus, the resolving power, x, is approximately equal to \(\lambda\).

In this demonstration we look at 1 micron-size latex spheres under a microscope. The spheres cannot be clearly resolved when viewed with a magnification of X1000. Rather, one sees spots of light surrounded by diffraction rings. Focusing in vain, the diffraction rings merely change from dark to light (or vice versa) as one passes through different regions of the Fresnel diffraction pattern.

Setting it up:  This is the same set-up as the Brownian Motion of Latex Speres demonstration. The microscope sits on a dedicated cart. A video camera displays the image. Use a well microscope slide¹ with a cover slip.

Comments:

1.  Globe Scientific Cavity Well Microscope Slides (VWR cat.no. 10118-600).